Integrating with partial fractions involves resolving an fractional integral into simpler fractions that can be integrated separately. Partial Fractions are the fractions that are formed when one single fraction is split into two or more simpler fractions.
consider the fraction:
$$\frac{5x-3}{(x+1)(x-3)} = \frac{2}{x+1} + \frac{3}{x-3} $$
As can be seen, the complex fraction on the left hand side has been split into two simpler equations as shown on the right hand right side.
To resolve a fraction into a partial fractions there are simple rules to be followed:
- If the degree of the numerator of a given fraction is equal to or greater than that of denominator, divide the numerator by the denominator until a reminder is obtained which is of lower degree than the denominator.
- To every linear factor like (X-A) in the denominator, there corresponds a partial fraction of the form:
$$\frac{A}{x-a}$$
3. To every repeated linear factor like (x-a)2 in the denominator their corresponds two partial fractions of the form:
$$\frac{A}{x-a} \ and \ \frac{B}{(x-a)^2}$$
similarly for factors like (x-a)3, then we have:
$$\frac{A}{x-a} \ \, \frac{B}{(x-a)^2} \ \ and \ \ \frac{C}{(x-a)^3}$$
4. To every quadratic factor like x2+ax + b, there corresponds partial fractions in the form:
$$\frac{cx+D}{x^2 + ax + b} $$
Repeated quadratic equation factors requires additional partial fractions as described in 3 above, thus a factor like (x2 + ax + b)2 would require:
$$\frac{CX+D}{x^2+ax+b} \ \ and \ \ \frac{Ex+F}{(x^2 + ax +b)^2}$$
Example problems in Integrating with partial fractions:
Resolve the following fraction into partial fraction:
$$\frac{x^3}{x^2-3x+2}$$
solution
if the numerator has more power or equal power to denominator , then you need to divide
Examples of such fractions includes:
$$\frac{x^2+2x+1}{x^2-3x+2}$$
and
$$\frac{x+1}{x+2}$$
The above two equations has the same power in both denominator and numerator.
Consider the fraction:
$$\frac{x^3}{x^2-3x+2}$$
We apply the long division as follow:
\begin{array}{r}
x\phantom{)} \\
x^2-3x+2{\overline{\smash{\big)}\,x^3 \phantom{)}}}\\
\end{array}
Taking the reading term in each ;
leading term in the numerator = x3
leading term is the term with the highest power in a polynomial expression
Remember:
$$\frac{x^3}{x^2} = x $$
similarly;
\begin{array}{r}
x+3\phantom{)} \\
x^2-3x+2{\overline{\smash{\big)}\,x^3+0x^2+0x+0\phantom{)}}}\\
\underline{ \ \phantom{}x^3-3x^2 +2x+0\phantom{-b}}\\
3x^2-2x+0\phantom{)}\\
\underline{-~\phantom{()}(3x^2-9x+6)}\\
7x-6\phantom{)}
\end{array}
The division of the polynomial gives 7x-6 as the remainder.
Therefore:
$$\frac{x^3}{x^2-3x+2}= (x+3) + \frac{7x-6}{x^2-3x+2}$$
The fraction
$$ \frac{7x-6}{x^2-3x+2}$$
Can be resolved into partial fraction using the expression:
$$\frac{7x-6}{x^2-3x+2} = \frac{7x-6}{(x-1)(x-2) }=\frac{A}{x-1} + \frac{B}{x-2} $$
We therefore have the expression:
$$\frac{A}{x-1} + \frac{B}{x-2} = \frac{A(x-2)+ B(x-1)}{(x-1)(x-2)}$$
opening the brackets on the numerator of the right hand side expression:
$$ \frac{7x-6}{(x-1)(x-2) }= \frac{Ax-2A+Bx-B}{(x-1)(x-2)} $$
Bringing like terms together:
7x-6 = Ax + Bx -2A -B
this means that:
$$7x= (A+B)x $$ and
$$-6 = -(2A+B)$$
Equating coefficients of x on both sides and the constants results to simultaneous equations:
$$A + B= 7 $$
$$2A+B = 6$$
solving the simultaneous equations we get that: A = -1 and B = 8
the equation:
$$\frac{7x-6}{x^2-3x+2} = \frac{7x-6}{(x-1)(x-2) }=\frac{A}{x-1} + \frac{B}{x-2} $$
becomes:
$$\frac{7x-6}{x^2-3x+2} =\frac{-1}{x-1} + \frac{8}{x-2} = \frac{8}{x-2} – \frac{1}{x-1}$$
hence:
$$\frac{x^3}{x^2-3x+2}= x+3 + \frac{8}{x-2} – \frac{1}{x-1} $$
Example
Resolve the fraction below into partial fractions:
$$\frac{4-2x}{(x^2+1)(x-1)^2}$$
solution
The highest power of x is 3 and hence we split the fraction into 3 smaller fraction such that:
$$\frac{4-2x}{(x^2+1)(x-1)^2} = \frac{Ax+B}{x^2+1} +\frac{C}{x-1}+\frac{D}{(x-1)^2}$$
$$\frac{4-2x}{(x^2+1)(x-1)^2}=\frac{(Ax+B)(x-1)^2 + C(x^2+1)(x-1)+D(x^2+1)}{(x^2+1)(x-1)^2 }$$
$${4-2x}={(Ax+B)(x-1)^2 + C(x^2+1)(x-1)+D(x^2+1)}$$
Expanding:
$$4-2x = Ax^3-2Ax^2+Ax+Bx^2-2Bx+B+Cx^3-Cx^2+Cx-C+Dx^2+D$$
Equating powers with same degree on both sides of the equation:
$$4-2x = Ax^3+Cx^3+Bx^2-2Ax^2-Cx^2+Dx^2+Ax-2Bx+Cx-C+D$$
$$4-2x =(A+B)x^3 + (B-2A-C+D)x^2+(A-2B+C)x-C+D$$
$$(A+C)x^3 = 0$$
$$(B-2A-C+D)x^2 = 0$$
$$(A-2B+C)x = -2x$$
$$B-C+D =4 $$
and hence we have the equations:
A+C = 0
B-2A-C+D = 0
A-2B+C = -2
B-C+D = 4
From the equations above we finds that:
A=2, B=1, C = -2, D=1
Therefore:
$$\frac{4-2x}{(x^2+1)(x-1)^2} = \frac{2x+1}{x^2+1} -\frac{2}{x-1}+\frac{1}{(x-1)^2}$$
Example 2: Integrating with partial fractions
Evaluate the integral:
$$\int \frac{5x-3}{(x+1)(x-3)} dx$$
solution
We resolve the complex fraction into partial fraction as follow:
$$\frac{5x-3}{(x+1)(x-3)} = \frac{A}{x+1} +\frac{B}{x-3} $$
$$\frac{A}{x+1} +\frac{B}{x-3} = \frac{A(x-3)+B(x+1)}{(x+1)(x-3)}$$
Therefore:
5x-3 = A(x-3) + B(x+1)=Ax-3A+Bx+B
5x-3 = A(x-3) + B(x+1)=Ax+Bx-3A+B
hence:
- A+B = 5
- -3A +B =-3
substituting for B in the second equation:
B = -3+3A
solving for B in the first equation:
A +(-3+3A) = 5
A-3+3A = 5
4A = 8
A=2
B = -3+3(2) = -3+6 = 3
hence our integral in partial fraction will become:
$$\int \frac{5x-3}{(x+1)(x-3)} dx = \int (\frac{2}{x+1}+\frac{3}{x-3})dx$$
$$\int \frac{5x-3}{(x+1)(x-3)} dx = \int \frac{2}{x+1}dx+\int \frac{3}{x-3}dx$$
but;
$$ \int \frac{2}{x+1}dx+\int \frac{3}{x-3}dx=2\int \frac{1}{x+1}dx+3\int \frac{1}{x-3}dx$$
and then using direct substitution:
$$2ln|x+1| + 3ln|x-3| + C$$
Example3: Integrating with partial fraction
$$\int \frac{4-2x}{(x^2+1)(x-1)^2}dx $$
Integrate:
solution
after resolving into partial fraction we get:
$$\frac{4-2x}{(x^2+1)(x-1)^2} = \frac{2x+1}{x^2+1}-\frac{2}{x-1} + \frac{1}{(x-1)^2}$$
The integral becomes:
$$\int \frac{4-2x}{(x^2+1)(x-1)^2}dx = \int \frac{2x+1}{x^2+1} dx- \int \frac{2}{x-1}dx + \int \frac{1}{(x-1)^2}dx$$
$$ =\int \frac{2x}{x^2+1}dx + \int \frac{1}{x^2+1} dx- 2\int \frac{1}{x-1}dx + \int \frac{1}{(x-1)^2}dx$$
consider the integral:
$$\int \frac{2x}{x^2+1}dx $$
using the substitution techniques:
u=x2+1
du/dx = 2x and hence du = 2xdx
$$\int \frac{2x}{u} \frac{du}{2x} = \int \frac{1}{u}du = lnu +c = ln|x^2+1|+c$$
also remember:
$$\int \frac{1}{x^2+1}dx = tan^{-1}(x) +c$$
$$2\int \frac{1}{x-1}dx= 2ln|x-1|+c $$
now we consider the integral:
$$\int \frac{1}{(x-1)^2}dx$$
let u =x-1; then du/dx = 1 and so du =dx
$$\int \frac{1}{U^2}du = \int u^{-2}du=\frac{u^{-1}}{-1}+c$$
but:
$$\frac{-1}{u} = \frac{-1}{x+1} +c $$
Therefore:
$$\int \frac{4-2x}{(x^2+1)(x-1)^2}dx =ln|x^2+1|+tan^{-1}(x)-2ln|x-1|-\frac{1}{x-1}+c$$
Example 4: Integrating with partial fractions
Evaluate:
$$\int \frac{x^2+1}{x^2-1}dx$$
solution
resolving the complex fraction into partial fractions we get:
$$ \frac{x^2+1}{x^2-1} = 1 + \frac{2}{x^2-1} = 1+\frac{2}{(x+1)(x-1)}$$
$$\frac{2}{(x+1)(x-1)} = \frac{A}{x+1}+\frac{B}{x-1}$$
2 = A(x-1)+B(x+1) = Ax- A+ Bx + B = Ax + Bx -A+B
A+B = 0 and -A+B = 2
A = -B
-(-B) +B = 2
2B =2 and so B=1
A=-1
Therefore:
$$\frac{2}{(x+1)(x-1)} = \frac{-1}{x+1} +\frac{1}{x-1} $$
$$ \frac{x^2+1}{x^2-1} = 1- \frac{1}{x+1} +\frac{1}{x-1} $$
hence
$$ \int \frac{x^2+1}{x^2-1} = \int (1- \frac{1}{x+1} +\frac{1}{x-1})dx $$
$$ = \int 1dx- \int \frac{1}{x+1} dx +\int \frac{1}{x-1})dx $$
$$=x-ln|x+1| + ln|x-1|+c$$
Revision Exercise
1. $$\int \frac{4x+5}{x^2+2x+2}$$
1. $$2ln|x^2+2x+2|+tan^{-1}(x+1)+c$$
2. $$\int \frac{x^3}{x-1}dx$$
2.$$\frac{1}{3}x^3+\frac{1}{2}x^2+x + ln|x-1|+c$$
3. $$ \int \frac{3-2x}{2x-1}dx $$
4. $$ \int \frac{dx}{(x-1)^2(x^2+1)}dx$$
4.$$ \frac{1}{2}ln|x-1| = \frac{1}{2(x-1)} + \frac{1}{4}ln|1+x^2|+c$$
5. $$\int \frac{5}{x^2+x-6}dx$$
5. $$ ln|\frac{x-2}{x+3}|+c$$
6.$$ \int \frac{7x^2+5}{(x+3)(x^2+4)} dx$$
6. $$2ln|x-3|-ln(x^2+4)+\frac{1}{2}tan^{-1}(\frac{x}{2})+c$$
Related Topics
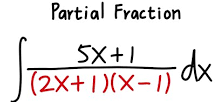

Leave a Reply