Linear magnification refers to the ratio of the size of an image to the size of the object that produces it, when viewed through an optical instrument like a microscope, telescope, or camera. In simpler terms, it’s how much larger or smaller the image appears compared to the actual object. For example, if a microscope makes an object appear 10 times larger than it actually is, the linear magnification is 10x.
This concept is crucial in optics and imaging to understand how instruments alter the apparent size of objects.
Formal definition of Linear Magnification
Linear Magnification M is defined as the ratio of the image height to object height
Magnification M = (height of image)/(height of object)
Suppose an object is positioned infront of a lenses as shown,

OB is the height of the object and IM is the height of the image.
If u is the object distance (PO) and v the image distance (PI), then triangle POB and PIM are similar
Hence using principles of similarities in triangles:
Therefore Magnification;
Example
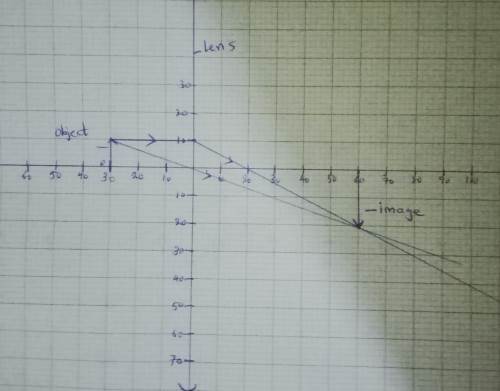
An object of height 10 cm is placed 30 infront of convex lens of focal length 20 cm. Use scale drawing to find position, size and nature of the image and Magnification.
solution
we use the scale of 1cm to represent 10cm horizontally and 1cm to represent 10cm vertically. The object is represented by an upright arrow that is placed 30cm on the principal axis from the line that represents the lens.

object distance = 30cm
image distance =60cm
height of image =20cm
height of object=10cm
The image is magnified as it is bigger than the object.
The image is position at 60cm which is beyond 2F on the other side of the lens. It is a real image.
Examination Questions
- Describe an experiment to determine the focal length of a concave mirror using a pin by no parallax method (3marks)
2. An object 5 cm high is placed 25cm from a convex lens of focal length 10 cm.
i. Draw a ray diagram on the grid provided (4 marks)
ii. From the ray diagram state the position of the image (1 mark)
iii. Determine the magnification of the image (1 mark)
3. Distinguish between refraction and dispersion of light


Leave a Reply