The table of tangents contains values for every acute angle from 0o to 90o. Each angle has a unique tangent ratio. This ratio is formed when two lines meet to make the angle.
Every combination of opposite and adjacent lines that makes a right angled triangle has a unique angle which they make.
If we know the acute angle in a right angled triangle, we can use tables of tangents. This helps us find its corresponding tangent ratio. Similarly, if we know the angle and just one side, we can find the angle’s ratio. Then, we use the tangent relationship to find the other side.
The table of tangents consists of angles of 0o to 90o expressed in 4 significant figures whose tangents has been recorded. All we need to do as mathematician is get a certain angle and find it’s corresponding ratio from the tables.
Angles in the table of tangents are expressed in degrees and points of degrees as well as in minutes. 1 degree (1o) is equivalent to 60 minutes(60′).
The table of tangents is divided into three major columns as shown in the table extract below:

The first column is the column with the column head labelled xo which represents whole number degrees from 0o to 90o .
The second column consists of 0.0o to 0.9o which divides a degree into 10 smaller units hence giving an accuracy of 0.1o.
The third column is the one labelled ADD column and provides the second decimal value of the angle. Using the table of tangents, we can find angles upto to second decimal places.
Example
Determine the tangent of 36.57o
solution
In the column labelled xo , look for the row headed 36 and then move along this row until you reach 0.5. The number at the intersection of 36 and 0.5 is 0.7400
note that the number is recorded as 7400 and not 0.7400. This is done to save on space but you should check the first column after 36, That is, column headed 0.0, whatever value that is stated on that row in that column should be used as the starting value for all the columns in that row.
so tan 36.5 =0.7400, to get the value for tan 36.57, we go to the add column and check on the column 0.07 and add it’s value on the far right of our previous value we read from the table. In this case it is 19 and should be read as 0.0019
hence tan 36.57 should be 0.7400+0.0019 = 0.7419
Example
Use tables to find the tangent of 77o48′
solution
1o=60′, hence 48′ = (48′ x 1o)/60′ = 0.8o
then 77o48′ can be expressed as 77.8o
From the tables, you identify row 77 at xo column then move up to to the column 0.8 and read off that value at the intersection. This value is 0.6252 hence tan 77o48′ = tan 77.8o = 0.6252
Example
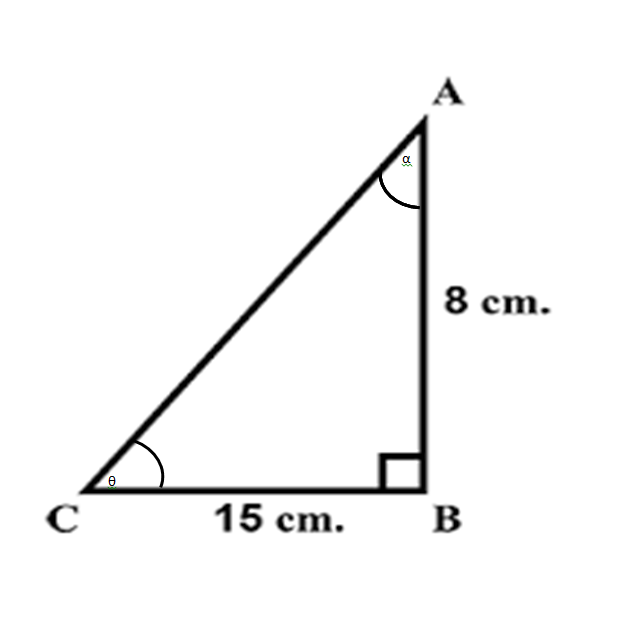
Find angle θ and α in the figure below.

Leave a Reply